Instabilities in viscoelastic flows are emerging as a rich class of fluid mechanical phenomena that are of much importance in polymer processing and coating operations. These instabilities are often found in nearly inertialess flows, and are driven solely by the non-Newtonian behavior of complex fluids such as polymer melts and solutions. However, most of the progress in the analysis of the stability of viscoelastic flows has been restricted to simple viscometric flows. The current analysis is based on finite element calculation of the steady base flows in complex geometries and the long-time solution of the time-dependent equations formed by linearization about the base flow. The equation set for the perturbations is solved either by direct time integration or by the Arnoldi method in which the most dangerous eigenvalues for the eigenvalue problem that corresponds to the long-time solution of the disturbance equations are determined. We also apply an energy analysis to examine poorly understood mechanisms of elastic instabilities in complex flows such as axisymmetric and planar contraction/expansion flows.

• P. Lu, P. Ochonma, R. Marthi, S.D. Prabhu, H. Asgar, Y.L. Joo, and G. Gadikota, “Preferential Formation of Uniform Spherical Vaterite by Harnessing Vortex Flows and Integrated CO2 Capture and Mineralization”, Chemical Engineering Journal 490, 151761 (2024).
• S. Zamani, J.S Won, M. Salim, M. AlAmer, C.W. Chang, P. Kumar, K. Amponsah, A.R. Lim, and Y.L. Joo, “Ultralight Graphene/Graphite Hybrid Fibers via Entirely Water-based Processes and Their Application to Density-controlled, High Performance Composites”, Carbon 173, 880–890 (2021).
• M. AlAmer, A.R. Lim, and Y.L. Joo, “Continuous Synthesis of Structurally Uniform Graphene Oxide Materials in a Model Taylor-Couette Flow Reactor”, Ind. Eng. Chem. Res.  58, 1123-1462 (2019).
• M.J. Divvela and Y.L. Joo, “Discretized Modeling of Beads on a String Morphology from Conducting and Viscoelastic Polymer Jets during Electrospinning”, J. of Applied Physics 121, 134306 (2017).
• E. Lee, D.L. Koch and and Y.L. Joo, “Cross Stream Forces and Velocities of Fixed and Freely Suspended Particles in Viscoelastic Plane Poiseuille Flow: Perturbation and Numerical Analysis”, Journal of Non-Newtonian Fluid Mechanics 165, 1309-1327 (2010).
• C.P. Carroll and Y.L. Joo, “Axisymmetric Instabilities in Electrospinning of Highly Conducting, Viscoelastic Polymer Solutions”, Physics of Fluids 21, 103101 (2009).
• C.P. Carroll and Y.L. Joo, “Axisymmetric Instabilities of Electrically Driven Viscoelastic Jets”, Journal of Non-Newtonian Fluid Mechanics, 153, 130-148 (2008).
• M.D. Smith, Y.L. Joo, R.C. Armstrong and R.A. Brown, “Linear Stability Analysis of Flow of an Oldroyd-B Fluid through a linear array of cylinders”, Journal of Non-Newtonian Fluid Mechanics, 109, 13-50 (2003)
• A. Caola, Y.L. Joo, R.C. Armstrong and R.A. Brown, “Highly Parallel Time Integration of Viscoelastic Flows”, Journal of Non-Newtonian Fluid Mechanics, 100, 191-216 (2001).
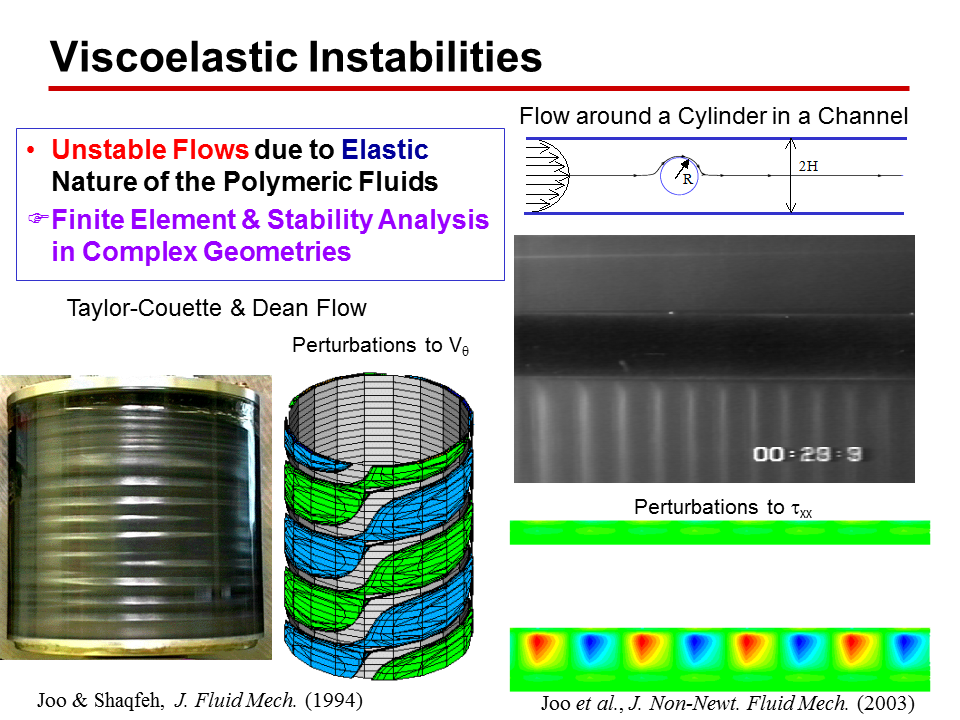
• Y.L. Joo and E.S.G. Shaqfeh, “Observations of Purely Elastic Instabilities in the Taylor-Dean Flow of a Boger Fluid” , Journal of Fluid Mechanics, 262, 27-73 (1994).
• Y.L. Joo and E.S.G. Shaqfeh, “The Effects of Inertia on the Viscoelastic Dean and Taylor-Couette Flow Instabilities with Application to Coating Flows” , Physics of Fluids A, 4, 2415–2431 (1992).
• Y.L. Joo and E.S.G. Shaqfeh “A Purely Elastic Instability in Dean and Taylor-Dean Flow” , Physics of Fluids A, 4, 524-543 (1992).
• Y.L. Joo and E.S.G. Shaqfeh, “Viscoelastic Poiseuille Flow Through a Curved Channel: A New Elastic Instability” , Physics of Fluids A, 3, 1691-1694 (1991).